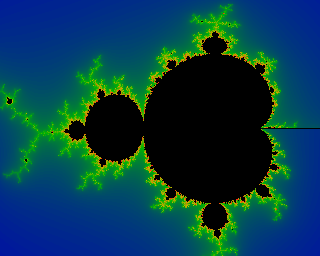
 In particular, can we find a self-similar, quasi-conformal mapping of the
M-set onto itself?
This image shows the result of one such mapping. Close, but no cigar.
To generate this image, we first 'flatten' the cardiod. Let
In particular, can we find a self-similar, quasi-conformal mapping of the
M-set onto itself?
This image shows the result of one such mapping. Close, but no cigar.
To generate this image, we first 'flatten' the cardiod. Let
c = r/2 eip (1 - r/2 eip)
The cardiod is then the straight line r=1. The angle p, we already know, can be used to determine the gaps. The gaps have a variety of self-similar mappings. For example:
p' = p / (1+p)
maps 1/2 to 1/3, and 1/3 to 1/4, and so on. That is, if p is the continued fraction p=[a1, a2,...] then a'1 = a1 + 1. Next, for good measure, we remap r' = r1/2 and use
c' = r'/2 eip' (1 - r'/2 eip')
to regain the cardiod shape. Because the gaps are self-similar, this shape is almost self-similar. The mystery question is: are there any better approximations, or even any exact remappings?. By the way, the west bud (now the 3-cycle bud) is not circular.
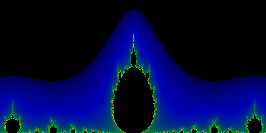 In this image, we use the map c = r/2 eip (1 - r/2 eip) with
r running from 1 at the bottom to 5 at the top, and p running from -1.1 pi to +1.1 pi
left to right. The cardiod maps to the area below r=1, but due to symmetry, it also
maps to the large bulb in the middle. (There are two solutions:
1/2 eit = r/2 eip, viz r=1, and the bulb in the middle, which is
1/2 eit = 1 - r/2 eip which is 0 = r2 - 4r cos p +3)
Anyway, we can see the buds nicely aligned along the r=1 line.
In this image, we use the map c = r/2 eip (1 - r/2 eip) with
r running from 1 at the bottom to 5 at the top, and p running from -1.1 pi to +1.1 pi
left to right. The cardiod maps to the area below r=1, but due to symmetry, it also
maps to the large bulb in the middle. (There are two solutions:
1/2 eit = r/2 eip, viz r=1, and the bulb in the middle, which is
1/2 eit = 1 - r/2 eip which is 0 = r2 - 4r cos p +3)
Anyway, we can see the buds nicely aligned along the r=1 line.
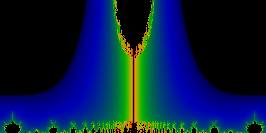 This image shows a rescaling: we magnify as we get to the middle. The map used here is
as above, but with r = 1+r' and r' = r'' / sin2 (p/2). We've essentially
discovered the asymptotic behaviour of the bud size: its easy to verify by visual
inspection that they are all the same height.
Recall that buds are located at p = 2pi/n for integer values of n. Also recall that
the 'antenna' indicates the n-cycle of the bud.
We can see from the above that this n is
the same n: viz, the 3-cycle bud is located at 2pi/3. Since sin(p/2)->p/2 for small p,
we have that the n-cycle bud on the main cardiod is about 1/n2 in size.
This image shows a rescaling: we magnify as we get to the middle. The map used here is
as above, but with r = 1+r' and r' = r'' / sin2 (p/2). We've essentially
discovered the asymptotic behaviour of the bud size: its easy to verify by visual
inspection that they are all the same height.
Recall that buds are located at p = 2pi/n for integer values of n. Also recall that
the 'antenna' indicates the n-cycle of the bud.
We can see from the above that this n is
the same n: viz, the 3-cycle bud is located at 2pi/3. Since sin(p/2)->p/2 for small p,
we have that the n-cycle bud on the main cardiod is about 1/n2 in size.
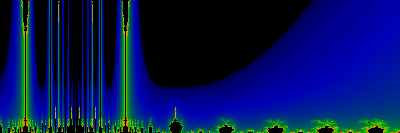 This image does that: along the x-axis, we have 2pi/p, and so the buds
are located at integer values.
This image does that: along the x-axis, we have 2pi/p, and so the buds
are located at integer values.
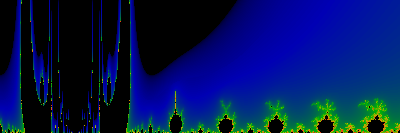 This image is as above, but with r' = r'' / (p/2) sin (p/2). (Note we've also scaled
the vertical axes for effect). Some very interesting shapes at n=1 and below.
Note that the n=2 bud is not symmetric, as it is above.
This image is as above, but with r' = r'' / (p/2) sin (p/2). (Note we've also scaled
the vertical axes for effect). Some very interesting shapes at n=1 and below.
Note that the n=2 bud is not symmetric, as it is above.
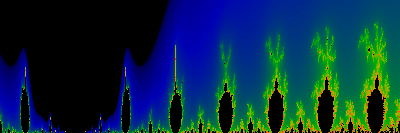 This image is as above, but with r' = r'' / (p/2)2 .
Once again, we've scale the vertical axes to help bring out some interesting details.
If the horizontal and vertical scale were the same, then the buds would be more circular.
And, once again, the n=2 bud is not symmetric, as it was earlier. On the other hand,
its much closer in size to the n=3 bud. We've traded size for symmetry.
This image is as above, but with r' = r'' / (p/2)2 .
Once again, we've scale the vertical axes to help bring out some interesting details.
If the horizontal and vertical scale were the same, then the buds would be more circular.
And, once again, the n=2 bud is not symmetric, as it was earlier. On the other hand,
its much closer in size to the n=3 bud. We've traded size for symmetry.




To contact Linas, see his Home Page.
